
mathworld.wolfram.com/vanderCorputSequence.html
Preview meta tags from the mathworld.wolfram.com website.
Linked Hostnames
5- 24 links tomathworld.wolfram.com
- 4 links towww.wolfram.com
- 3 links towww.wolframalpha.com
- 1 link towolframalpha.com
- 1 link towww.amazon.com
Thumbnail
Search Engine Appearance
van der Corput Sequence -- from Wolfram MathWorld
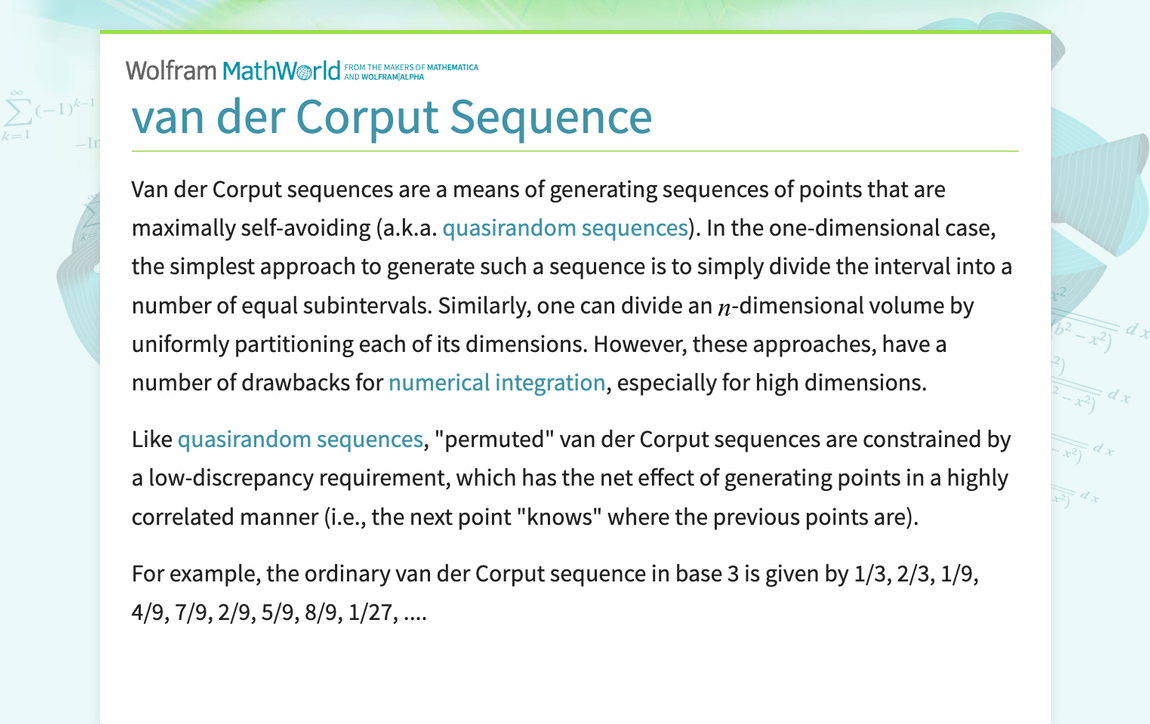
Van der Corput sequences are a means of generating sequences of points that are maximally self-avoiding (a.k.a. quasirandom sequences). In the one-dimensional case, the simplest approach to generate such a sequence is to simply divide the interval into a number of equal subintervals. Similarly, one can divide an n-dimensional volume by uniformly partitioning each of its dimensions. However, these approaches, have a number of drawbacks for numerical integration, especially for high...
Bing
van der Corput Sequence -- from Wolfram MathWorld
Van der Corput sequences are a means of generating sequences of points that are maximally self-avoiding (a.k.a. quasirandom sequences). In the one-dimensional case, the simplest approach to generate such a sequence is to simply divide the interval into a number of equal subintervals. Similarly, one can divide an n-dimensional volume by uniformly partitioning each of its dimensions. However, these approaches, have a number of drawbacks for numerical integration, especially for high...
DuckDuckGo
van der Corput Sequence -- from Wolfram MathWorld
Van der Corput sequences are a means of generating sequences of points that are maximally self-avoiding (a.k.a. quasirandom sequences). In the one-dimensional case, the simplest approach to generate such a sequence is to simply divide the interval into a number of equal subintervals. Similarly, one can divide an n-dimensional volume by uniformly partitioning each of its dimensions. However, these approaches, have a number of drawbacks for numerical integration, especially for high...
General Meta Tags
20- titlevan der Corput Sequence -- from Wolfram MathWorld
- DC.Titlevan der Corput Sequence
- DC.CreatorWeisstein, Eric W.
- DC.DescriptionVan der Corput sequences are a means of generating sequences of points that are maximally self-avoiding (a.k.a. quasirandom sequences). In the one-dimensional case, the simplest approach to generate such a sequence is to simply divide the interval into a number of equal subintervals. Similarly, one can divide an n-dimensional volume by uniformly partitioning each of its dimensions. However, these approaches, have a number of drawbacks for numerical integration, especially for high...
- descriptionVan der Corput sequences are a means of generating sequences of points that are maximally self-avoiding (a.k.a. quasirandom sequences). In the one-dimensional case, the simplest approach to generate such a sequence is to simply divide the interval into a number of equal subintervals. Similarly, one can divide an n-dimensional volume by uniformly partitioning each of its dimensions. However, these approaches, have a number of drawbacks for numerical integration, especially for high...
Open Graph Meta Tags
5- og:imagehttps://mathworld.wolfram.com/images/socialmedia/share/ogimage_vanderCorputSequence.png
- og:urlhttps://mathworld.wolfram.com/vanderCorputSequence.html
- og:typewebsite
- og:titlevan der Corput Sequence -- from Wolfram MathWorld
- og:descriptionVan der Corput sequences are a means of generating sequences of points that are maximally self-avoiding (a.k.a. quasirandom sequences). In the one-dimensional case, the simplest approach to generate such a sequence is to simply divide the interval into a number of equal subintervals. Similarly, one can divide an n-dimensional volume by uniformly partitioning each of its dimensions. However, these approaches, have a number of drawbacks for numerical integration, especially for high...
Twitter Meta Tags
5- twitter:cardsummary_large_image
- twitter:site@WolframResearch
- twitter:titlevan der Corput Sequence -- from Wolfram MathWorld
- twitter:descriptionVan der Corput sequences are a means of generating sequences of points that are maximally self-avoiding (a.k.a. quasirandom sequences). In the one-dimensional case, the simplest approach to generate such a sequence is to simply divide the interval into a number of equal subintervals. Similarly, one can divide an n-dimensional volume by uniformly partitioning each of its dimensions. However, these approaches, have a number of drawbacks for numerical integration, especially for high...
- twitter:image:srchttps://mathworld.wolfram.com/images/socialmedia/share/ogimage_vanderCorputSequence.png
Link Tags
4- canonicalhttps://mathworld.wolfram.com/vanderCorputSequence.html
- preload//www.wolframcdn.com/fonts/source-sans-pro/1.0/global.css
- stylesheet/css/styles.css
- stylesheet/common/js/c2c/1.0/WolframC2CGui.css.en
Links
33- http://www.wolframalpha.com/input/?i=grad+sqrt%28r%29+cos%28theta%29
- https://mathworld.wolfram.com
- https://mathworld.wolfram.com/NumericalIntegration.html
- https://mathworld.wolfram.com/QuasirandomSequence.html
- https://mathworld.wolfram.com/about