mathworld.wolfram.com/Euler-LagrangeDifferentialEquation.html

Preview meta tags from the mathworld.wolfram.com website.

Linked Hostnames

6

Thumbnail

Search Engine Appearance

Google

https://mathworld.wolfram.com/Euler-LagrangeDifferentialEquation.html

Euler-Lagrange Differential Equation -- from Wolfram MathWorld

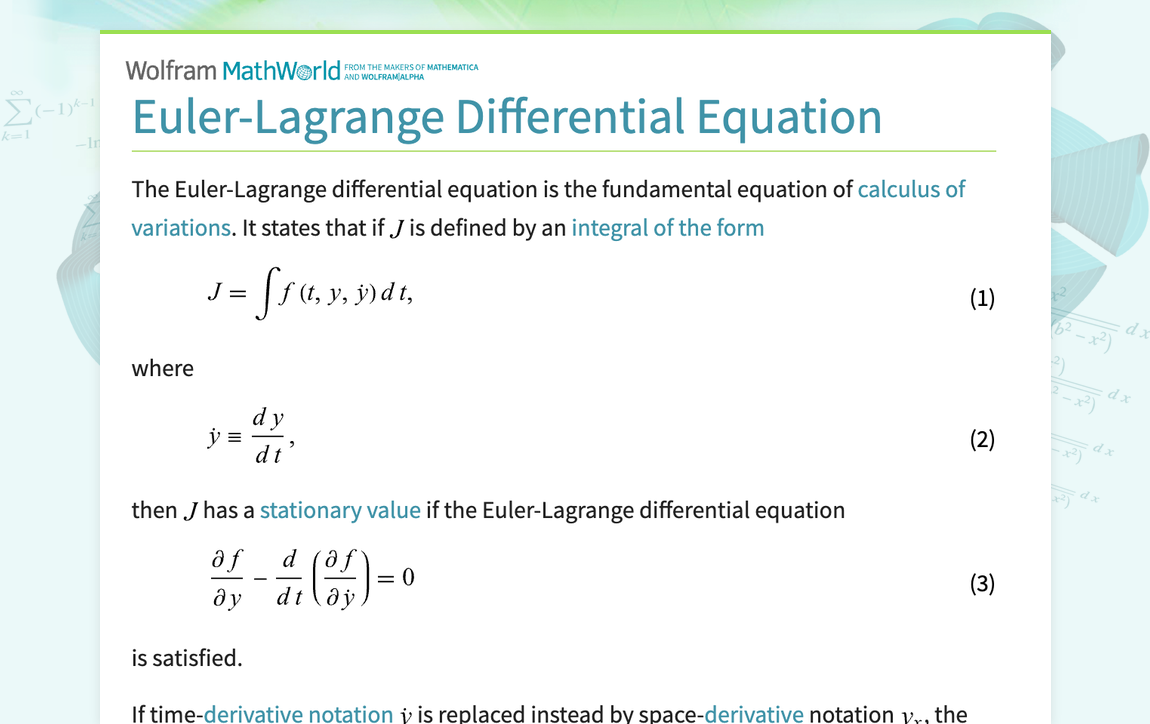
The Euler-Lagrange differential equation is the fundamental equation of calculus of variations. It states that if J is defined by an integral of the form J=intf(t,y,y^.)dt, (1) where y^.=(dy)/(dt), (2) then J has a stationary value if the Euler-Lagrange differential equation (partialf)/(partialy)-d/(dt)((partialf)/(partialy^.))=0 (3) is satisfied. If time-derivative notation y^. is replaced instead by space-derivative notation y_x, the equation becomes ...



Bing

Euler-Lagrange Differential Equation -- from Wolfram MathWorld

https://mathworld.wolfram.com/Euler-LagrangeDifferentialEquation.html

The Euler-Lagrange differential equation is the fundamental equation of calculus of variations. It states that if J is defined by an integral of the form J=intf(t,y,y^.)dt, (1) where y^.=(dy)/(dt), (2) then J has a stationary value if the Euler-Lagrange differential equation (partialf)/(partialy)-d/(dt)((partialf)/(partialy^.))=0 (3) is satisfied. If time-derivative notation y^. is replaced instead by space-derivative notation y_x, the equation becomes ...



DuckDuckGo

https://mathworld.wolfram.com/Euler-LagrangeDifferentialEquation.html

Euler-Lagrange Differential Equation -- from Wolfram MathWorld

The Euler-Lagrange differential equation is the fundamental equation of calculus of variations. It states that if J is defined by an integral of the form J=intf(t,y,y^.)dt, (1) where y^.=(dy)/(dt), (2) then J has a stationary value if the Euler-Lagrange differential equation (partialf)/(partialy)-d/(dt)((partialf)/(partialy^.))=0 (3) is satisfied. If time-derivative notation y^. is replaced instead by space-derivative notation y_x, the equation becomes ...

  • General Meta Tags

    23
    • title
      Euler-Lagrange Differential Equation -- from Wolfram MathWorld
    • DC.Title
      Euler-Lagrange Differential Equation
    • DC.Creator
      Weisstein, Eric W.
    • DC.Description
      The Euler-Lagrange differential equation is the fundamental equation of calculus of variations. It states that if J is defined by an integral of the form J=intf(t,y,y^.)dt, (1) where y^.=(dy)/(dt), (2) then J has a stationary value if the Euler-Lagrange differential equation (partialf)/(partialy)-d/(dt)((partialf)/(partialy^.))=0 (3) is satisfied. If time-derivative notation y^. is replaced instead by space-derivative notation y_x, the equation becomes ...
    • description
      The Euler-Lagrange differential equation is the fundamental equation of calculus of variations. It states that if J is defined by an integral of the form J=intf(t,y,y^.)dt, (1) where y^.=(dy)/(dt), (2) then J has a stationary value if the Euler-Lagrange differential equation (partialf)/(partialy)-d/(dt)((partialf)/(partialy^.))=0 (3) is satisfied. If time-derivative notation y^. is replaced instead by space-derivative notation y_x, the equation becomes ...

  • Open Graph Meta Tags

    5
    • og:image
      https://mathworld.wolfram.com/images/socialmedia/share/ogimage_Euler-LagrangeDifferentialEquation.png
    • og:url
      https://mathworld.wolfram.com/Euler-LagrangeDifferentialEquation.html
    • og:type
      website
    • og:title
      Euler-Lagrange Differential Equation -- from Wolfram MathWorld
    • og:description
      The Euler-Lagrange differential equation is the fundamental equation of calculus of variations. It states that if J is defined by an integral of the form J=intf(t,y,y^.)dt, (1) where y^.=(dy)/(dt), (2) then J has a stationary value if the Euler-Lagrange differential equation (partialf)/(partialy)-d/(dt)((partialf)/(partialy^.))=0 (3) is satisfied. If time-derivative notation y^. is replaced instead by space-derivative notation y_x, the equation becomes ...

  • Twitter Meta Tags

    5
    • twitter:card
      summary_large_image
    • twitter:site
      @WolframResearch
    • twitter:title
      Euler-Lagrange Differential Equation -- from Wolfram MathWorld
    • twitter:description
      The Euler-Lagrange differential equation is the fundamental equation of calculus of variations. It states that if J is defined by an integral of the form J=intf(t,y,y^.)dt, (1) where y^.=(dy)/(dt), (2) then J has a stationary value if the Euler-Lagrange differential equation (partialf)/(partialy)-d/(dt)((partialf)/(partialy^.))=0 (3) is satisfied. If time-derivative notation y^. is replaced instead by space-derivative notation y_x, the equation becomes ...
    • twitter:image:src
      https://mathworld.wolfram.com/images/socialmedia/share/ogimage_Euler-LagrangeDifferentialEquation.png

  • Link Tags

    4
    • canonical
      https://mathworld.wolfram.com/Euler-LagrangeDifferentialEquation.html
    • preload
      //www.wolframcdn.com/fonts/source-sans-pro/1.0/global.css
    • stylesheet
      /css/styles.css
    • stylesheet
      /common/js/c2c/1.0/WolframC2CGui.css.en

Links

53